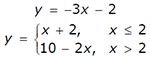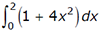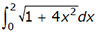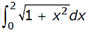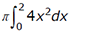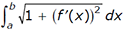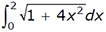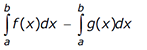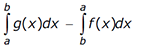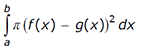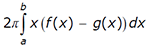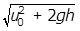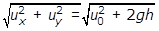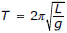Skip to main content

Preparation Manual

Print this page

Section 4: Sample Selected-Response Questions
Physics/Mathematics 7–12 (243)

Expand All Answers | Collapse All Answers

This section presents some sample exam questions for you to review as part of your preparation for the exam. To demonstrate how each competency may be assessed, sample questions are accompanied by the competency that they measure. While studying, you may wish to read the competency before and after you consider each sample question. Please note that the competency statements do not appear on the actual exam.

For each sample exam question, there is a correct answer and a rationale for each answer option. The sample questions are included to illustrate the formats and types of questions you will see on the exam; however, your performance on the sample questions should not be viewed as a predictor of your performance on the actual exam.

The following reference materials will be available to you during the exam:

Domain I—Number Concepts

Competency 001—The teacher understands the real number system and its structure, operations, algorithms and representations.

1. A light year is the distance light travels in one year. The star Procyon is approximately 1.08 times 10 to the power of 17 meters from our Sun. If light travels at 3.00 times 10 to the power of 8 meters per second, and if there are approximately 3.16 times 10 to the power of 7 seconds in a year, what is the approximate distance, in light years, from Procyon to our Sun?

  1. 11.39
  2. 87.78
  3. 1.14 times 10 squared
  4. 8.78 times 10 to the power of negative 2)
Enter to expand or collapse answer.Answer expanded
Option A is correct because the distance light travels in one year is open paren 3.00 times 10 to the power of 8 m/sec close paren open paren 3.16 times 10 to the power of 7 sec/yr close paren equals 9.48 times 10 to the power of 15 m/yr. Therefore the distance from the star to our sun is open paren 1.08 times 10 to the power of 17 m close paren divided by open paren 9.48 times 10 to the power of 15 m/yr close paren is approximately 11.39 light-years. Options B and D are incorrect because the calculation open paren 9.48 times 10 to the power of 15 m/yr close paren divided by open paren 1.08 times 10 to the power of 17 m close paren and 8.78 times 10 negative squared have units of 1 over year instead of the correct unit years. Option C is incorrect because 0.114 times 10 squared is not equivalent to 1.14 times 10 squared.

2. The number of bacteria in a colony increases from 400 to 800 during 1 hour. What is the rate of growth?

  1. 400% per hour
  2. 200% per hour
  3. 100% per hour
  4. 50% per hour
Enter to expand or collapse answer.Answer expanded
Option C is correct because the growth rate in 1 hour is open paren 800 minus 400 over 400 close paren times 100 equals start fraction 400 over 400 end fraction times 100 equals 100%. Option A is incorrect because the growth is 400 bacteria per hour, not 400%. Options B and D are incorrect because these growth rates are not supported by the data.
Competency 002—The teacher understands the complex number system and its structure, operations, algorithms and representations.

Use the figure below to answer the question that follows.

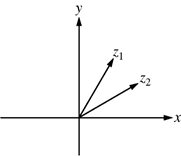
The figure shows two vectors, labeled z sub 1 and z sub 2, in a complex x, y plane.

Both start at the origin and extend up to the right. They appear to be the same length. The angles from the y axis to z sub 1, from z sub 1 to z sub 2, and from z sub 2 to the x axis appear to be roughly the same.

3. Two complex numbers, Z sub 1 and Z sub 2 are shown in the complex plane above. Which of the following graphs could depict the product Z sub 1 Z sub 2 in the complex plane?

Each response shows the same diagram with the addition of a third vector, labeled z sub 1 z sub 2.

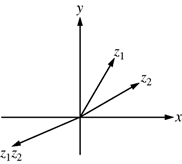  1. diagram response Az sub 1 z sub 2 starts at the origin and points down to the left. The angle between z sub 1 z sub 2 and the x axis is slightly less than the angle between z sub 2 and the x axis. It is about the same length as z sub 1 and z sub 2.
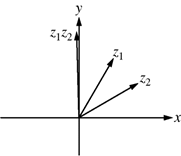  2. diagram response Bz sub 1 z sub 2 starts at the origin and points up to the right. The angle between z sub 1 z sub 2 and the y axis is very small. It is about half again as long as z sub 1 and z sub 2.
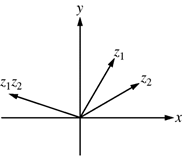  3. diagram response Cz sub 1 z sub 2 starts at the origin and points up to the left. The angle between z sub 1 z sub 2 and the x axis is somewhat less than the angle between z sub 2 and the x axis. It is a little longer than as z sub 1 and z sub 2.
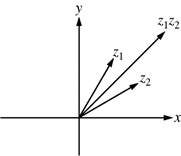  4. diagram response Dz sub 1 z sub 2 starts at the origin and points up to the right about halfway between z sub 1 and z sub 2. It appears to be twice as long as z sub 1 and z sub 2.
Enter to expand or collapse answer.Answer expanded
Option B is correct because the argument of the product of two complex numbers is the sum of their arguments. The sum of the arguments of the two given complex numbers is slightly more than pi over 2 radians. Only option B has the correct argument for the product. Options A, C and D are incorrect because the arguments of Z sub 1 Z sub 2 shown are not the sum of the arguments of Z sub 1 and Z sub 2.
Competency 003—The teacher understands number theory concepts and principles and uses numbers to model and solve problems in a variety of situations.

4. Given that n minus 5 and n plus 6 are positive integers both divisible by prime number p, which of the following integers must also be divisible by p?

  1. n plus 1
  2. n minus 30
  3. n plus 28
  4. n minus 11
Enter to expand or collapse answer.Answer expanded
Option C is correct because p divides both n minus 5 and n plus 6, therefore p must also divide their difference, open paren n plus 6 close paren minus open paren n minus 5 close paren equal 11, and thus p equals 11. Then p is also a factor of n plus 6 plus 11k and n minus 5 plus 11k for any integer k. Of the given options, only n plus 28 equals n plus 6 plus 22 equals n minus 5 plus 33 has this form. Options A, B and D are incorrect because these integers cannot be written in the form n plus 6 plus 11k or n minus 5 plus 11k, for some integer k.

5. On the first day of 2011 Asad deposited $500 into a savings account earning 3 percent annual interest, compounded annually at the end of the year. Asad made no additional deposits or withdrawals from the account during the year. On the first day of 2012, Asad deposited an additional $500 into the account, earning the same annual interest, compounded annually. Asad made no additional deposits or withdrawals from the account during the year. On the first day of 2013, Asad deposited an additional $500 into the account, earning the same annual interest, compounded annually. If no additional deposits or withdrawals were made, which of the following expressions represents the amount of money in Asad's account at the end of 2013?

  1. 3 open bracket 500 plus 500 open paren 0.03 close paren close bracket plus 500 open paren 0.06 close paren
  2. 3 open bracket 500 plus 500 open paren 1.03 close paren plus 500 open paren 2.06 close paren close bracket
  3. 500 open paren 1.03 close paren plus 500 open paren 1.03 close paren squared plus 500 open paren 1.03 close paren cubed
  4. 500 open paren 0.03 close paren plus 500 open paren 0.03 close paren squared plus 500 open paren 0.03 close paren cubed
Enter to expand or collapse answer.Answer expanded
Option C is correct because the first term represents the value of the third deposit, the second term represents the value of the second deposit after two years and the third term represents the value of the first deposit after three years. The sum of these terms gives the total amount of money in the account at the end of the third year. Options A and B are incorrect because the compounding of the interest is not correct. Option D is incorrect because the terms 500 open paren 0.03 close paren give the value of the interest, not the principal and interest.

Domain II—Patterns and Algebra

Competency 007—The teacher understands polynomial, rational, radical, absolute value and piecewise functions, analyzes their algebraic and graphical properties and uses them to model and solve problems.

Use the equations below to answer the question that follows.

first equation y equals negative 3x minus 2 over second equation y equals left curly bracket line 1 x plus 2 comma x is less than or equal to 2 line 2 10 minus 2x comma x is greater than 2

6. Which of the following are the points of intersection of the graphs in the xy-plane of the equations shown?

  1. (negative 1, 1) only
  2. (negative 1, negative 5) only
  3. (negative 12, 34) only
  4. (negative 1, 1) and (negative 12, 34)
Enter to expand or collapse answer.Answer expanded
Option A is correct because the graph of the line y equals negative 3x minus 2 intersects the graph of y equals x plus 2 at the point (negative 1, 1), and negative 1 is less than 2. The graph of the line y equals negative 3x minus 2 intersects the line y equals 10 minus 2x at the point (negative 12, 34), but this point is not on the graph of the piecewise defined function because negative 12 is not greater than 2. Option B is incorrect because the graph of the line y equals negative 3x minus 2 does not intersect the graph of y equals x plus 2 at the point (negative 1, negative 5). Option C is incorrect because the graph of the line y equals negative 3x minus 2 intersects the line y equals 10 minus 2x at the point (negative 12, 34), but this point is not on the graph of the piecewise defined function because negative 12 is not greater than 2. Option D is incorrect because there is only one point of intersection between the two graphs.
Competency 008—The teacher understands exponential and logarithmic functions, analyzes their algebraic and graphical properties and uses them to model and solve problems.

7. Which of the following is a vertical asymptote of the graph of y equals 3 plus log subscript 2 open paren x minus 4 close paren?

  1. y equals 3
  2. y equals negative 4
  3. x equals negative 4
  4. x equals 4
Enter to expand or collapse answer.Answer expanded
Option D is correct because the graph of y equals log subscript 2 baseline x has a vertical asymptote of the line x equals 0, the y-axis. The function y equals 3 plus log subscript 2 open paren x minus 4 close paren is a transformation of the graph of y equals log subscript 2 baseline x, with a vertical shift of 3 and a horizontal shift of 4. Thus, the vertical asymptote shifts horizontally from x equals 0 to x equals 4. Options A and B are incorrect because the lines y equals 3 and y equals negative 4 are horizontal and cannot be vertical asymptotes. Option C is incorrect because the graph of y equals log subscript 2 baseline x is shifted horizontally by the transformation to the right, not the left.
Competency 010—The teacher understands and solves problems using differential and integral calculus.

8. d over dx integral 0 to x sine t cosine t dt equals

  1. sine squared x cosine squared x over 4
  2. cosine 2 x
  3. sine squared x over 2
  4. sine x cosine x
Enter to expand or collapse answer.Answer expanded
Option D is correct because this is a direct application of the Fundamental Theorem of Calculus. Options A, B and C are incorrect because these are incorrect applications of the formulas for finding derivatives and calculating integrals.

Domain III—Geometry and Measurement

Competency 011—The teacher understands measurement as a process.

9. Which of the following definite integrals represents the length of the curve y equals x squared plus 1 between the points (0, 1) and (2, 5)?

  1. integral 0 to 2 open paren 1 plus 4x squared close paren dx
  2. integral 0 to 2 square root of 1 plus 4x squared dx
  3. integral 0 to 2 square root of 1 plus x squared dx
  4. pi integral 0 to 2 4x squared dx
Enter to expand or collapse answer.Answer expanded
Option B is correct because the length of the curve y equals f of x between x equals a and x equals b is found by the integral the integral of a to b square root 1 plus (f prime of x) squared dx. Thus, because y prime equals 2x, the length of the curve is the integral of 0 to 2 the square root of 1 plus 4x squared over this interval. Options A, C and D are incorrect because they are not correct applications of the length of a curve formula.

Use the figure below to answer the question that follows.

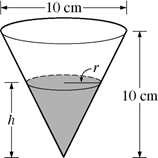
The figure shows a conical container with the point down.

The diameter at the top is dimensioned at 10 cm. The height is dimensioned as 10 cm. The container is partially filled with water, to a depth dimensioned as h. A radial line on the surface of the water is labeled r.

10. An inverted circular cone contains water, as shown. If the height, h, of the water is 8 centimeters, what is the radius, r, of the surface of the water, in centimeters?

  1. 8
  2. 6
  3. 4
  4. 2
Enter to expand or collapse answer.Answer expanded
Option C is correct because the ratio of r to h is equal to the ratio of the radius of the cone to the height of the cone, by the similarity of triangles. Because the radius of the cone is 10 over 2 equals 5, and r over 8 equals 5 over 10, then r equals 4. Options A, B and D are incorrect because a radius of these lengths would not satisfy the required ratio.

Use the figure below to answer the question that follows.

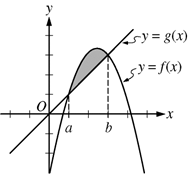
The figure shows two functions plotted on an x, y plane.

The axes have regularly spaced tick marks with no numerical values indicated. The first positive x tick mark is labeled A, and the third positive x tick mark is labeled b. There is a parabolic curve labeled y = f of x. It starts at the y axis three tick marks below the x axis, curves up to the right through the x axis before the first tick mark, reaches a peak y value about halfway between the third and fourth tick marks on the y axis and about halfway between the second and third tick marks on the x axis, then slopes down through the x axis just after the fourth tick mark. There is also a straight line labeled y = g of x. It passes from the lower left quadrant, through the origin, through the f of x curve at an x value of A, through the f of x curve again at an x value of b, then on up to the right. The area under the peak of the f of x curve, between the curve and the g of x line, is shaded.

11. Let f and g be continuous functions defined on the interval [a, b], as shown. Which of the following can be used to find the area of the shaded region?

  1. the integral from A to b of f of x dx, minus the integral from A to b of g of x dx.
  2. the integral from A to b of g of x dx, minus the integral from b to A of f of x dx.
  3. the integral from A to b of π times open paren f of x minus g of x, close paren, squared, dx.
  4. 2 pi the integral from A to b of x times open paren f of x minus g of x, close paren, dx.
Enter to expand or collapse answer.Answer expanded
Option A is correct because the shaded area is found by subtracting the area under the graph of g from a to b, 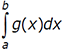the integral from A to b of g of x dx, from the area under the graph of f from a to b, 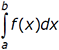the integral from A to b of f of x dx. Option B is incorrect because the expression yields the negative of the area of the shaded region. Option C is incorrect because the expression represents the volume of the solid of revolution generated by revolving the shaded region about the x-axis. Option D is incorrect because the expression represents the volume of the solid of revolution generated by revolving the shaded region about the y-axis.
Competency 013—The teacher understands the results, uses and applications of Euclidian geometry.

Use the figure below to answer the question that follows.

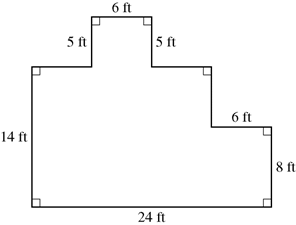
The figure shows a rectilinear shape with numerous sides

From the bottom left corner, proceeding counter clockwise, the distances from one corner to another are as follows. 24 feet to the right. 8 feet up. 6 feet to the left. an unspecified distance to the left. 5 feet up. 6 feet to the left. 5 feet down. an unspecified distance to the left. 14 feet down, which returns to the starting point.

12. The floor plan of Nick's den is shown. Nick wishes to purchase square tiles that are 12 inches on each side to tile the entire floor. To allow for possible breakage, he plans to purchase more tiles to cover an additional 10 percent of the floor area. How many tiles will Nick purchase?

  1. 330 tiles
  2. 363 tiles
  3. 366 tiles
  4. 402 tiles
Enter to expand or collapse answer.Answer expanded
Option B is correct because the floor area consists of three rectangles, 8 times 24, 6 times 18, and 5 times 6, for a total area of 330 square feet. The tiles are each 1 square foot. Allowing for 10 percent breakage, Nick needs to purchase an additional 33 equals 0.10 open paren 330 close paren tiles for a total of 363 tiles. Option A is incorrect because 330 square feet is the total area, and does not include extra tiles required in case of breakage. Option C is incorrect because 366 is the incorrect area derived from 14 times 24 plus 5 times 6. Option D is incorrect because 402 is derived from the incorrect area of 366, plus 10 percent.
Competency 014—The teacher understands coordinate, transformational and vector geometry and their connections.

13. Let the vectors i and j be given by i = (1, 0) and j = (0, 1). A person walks 80 feet due east, and then turns counter-clockwise 40 degrees and walks an additional 60 feet in that direction. Which of the following vectors describes the person's final position relative to his or her starting position?

  1. open paren 60 cosine ⁡40 degrees close paren i plus open paren 80 plus 60 cosine 40 degrees close paren j
  2. open paren 60 cosine 40 degrees close paren i plus open paren 80 plus 60 sin⁡e 40 degrees close paren j
  3. open paren 80 plus 60 sin⁡e 40 degrees close paren i plus open paren 60 sine ⁡40 degrees close paren j
  4. open paren 80 plus 60 cosine 40 degrees close paren i plus open paren 60 sin⁡e 40 degrees close paren j
Enter to expand or collapse answer.Answer expanded
Option D is correct because the first vector expressed in component form is 80i and the second vector is open paren 60 cosine 40 degrees close paren i plus open paren 60 sin⁡e 40 degrees close paren j. The resultant is then open paren 80 plus 60 cosin⁡e 40 degrees close paren i plus open paren 60 sine ⁡40 degrees close paren j. Options A and B are incorrect because the component 80j would result from walking due north rather than walking due east. Option C is incorrect because the second direction vector is open paren 60 cosine 40 degrees close paren i plus open paren 60 sin⁡e 40 degrees close paren j not open paren 60 sine 40 degrees close paren i plus open paren 60 sin⁡e 40 degrees close paren j.

Domain IV—Probability and Statistics

Competency 015—The teacher understands how to use appropriate graphical and numerical techniques to explore data, characterize patterns and describe departures from patterns.

Use the chart below to answer the question that follows.

SALARIES OF STUDENTS WORKING PART-TIME

The chart is a bar graph titled Salaries of Students Working Part-Time.

The vertical axis is labeled Number of Students and has values marked from 0 to 25 in increments of 5. The horizontal axis is labeled Weekly Salaries and has values marked as $0–$99, $100–$199, $200–$299, $300–$399, and $400–$499. The data bar values are as follows: Weekly Salary, $0–$99, 10 students. Weekly Salary, $100–$199, 18 students. Weekly Salary, $200–$299, 20 students. Weekly Salary, $300–$399, 8 students. Weekly Salary, $400–$499, 5 students.

14. The bar graph shown represents the distributions of weekly salaries, rounded to the nearest dollar, for a group of students with part-time jobs. Which of the following statements is true?

  1. The range of the weekly salaries is 499 dollars.
  2. The median weekly salary is between 240 dollars and 260 dollars.
  3. The average weekly salary is between 167 dollars and 267 dollars.
  4. The mode of the weekly salaries is between 200 dollars and 299 dollars.
Enter to expand or collapse answer.Answer expanded
Option C is correct because the chart shown is a histogram, and each bar displays a range of salaries. The chart shows that there are salaries of 61 students recorded. To calculate the possible average salary, find the weighted mean of the lowest possible salary and the highest possible salary, as shown in the chart. The weighted mean of the lowest possible salary is

10 open paren 0 close paren plus 18 open paren 100 close paren plus 20 open paren 200 close paren plus 8 open paren 300 close paren plus 5 open paren 400 close paren over 61 equals 10,200 over 61 equals 167.21 dollars

The weighted mean of the highest possible salary is

10 open paren 99 close paren plus 18 open paren 199 close paren plus 20 open paren 299 close paren plus 8 open paren 399 close paren plus 5 open paren 499 close paren over 61 equals 16,239 over 61 equals 266.21 dollars

Thus, the average weekly salary is greater than 167 dollars and less than 267 dollars. Option A is incorrect because the range of the salaries cannot be determined from the information given; the highest and lowest salaries are not indicated on the chart. Option B is incorrect because the median salary is determined by ordering the salaries from least to greatest and finding the salary in the middle. There are 61 salaries, so the 31st salary is the median and will lie in the bar labeled 200 dollars to 299 dollars. Option D is incorrect because the mode is the salary that occurs most often in the list of salaries, but the individual values are not provided.
Competency 016—The teacher understands concepts and applications of probability.

15. Mr. Allen's math class consists of 15 boys and 15 girls. Each day he randomly selects 3 students to present homework problems on the blackboard. What is the probability that the three chosen students will be all boys? (Note: n choose r equals n factorial over open paren n minus r close paren factorial r factorial denotes combinations of n objects taken r at a time, and n permutation r equals n factorial over open paren n minus r close paren factorial denotes permutations of n objects taken r at a time.)

  1. 15 permutation 3 over 30 choose 3
  2. 15 choose 3 over 15 permutation 3
  3. 15 choose 3 over 30 choose 3
  4. 15 permutation 3 over 15 choose 3
Enter to expand or collapse answer.Answer expanded
Option C is correct because 30 choose 3 gives the number of ways to choose 3 students from the 30-person class, and 15 choose 3 gives the number of ways of choosing 3 boys from among the 15 boys. Thus, the ratio 15 choose 3 over 30 choose 3 gives the probability that the teacher will choose 3 boys. Options A, B and D are incorrect because the numbers given do not correctly count the number of students to select.
Competency 017—The teacher understands the relationships among probability theory, sampling and statistical inference, and how statistical inference is used in making and evaluating predictions.

16. A packing company packages approximately 10,000 packages of noodles each day. The packages have a mean weight of 16 ounces with a standard deviation of 0.2 ounces. An inspector selects multiple samples of 25 packages each and computes the mean weight of each sample. Which of the following is the best estimate of the standard deviation of the mean weights of the samples?

  1. 0.02
  2. 0.04
  3. 0.2
  4. 1.0
Enter to expand or collapse answer.Answer expanded
Option B is correct because the standard deviation of the sample means should be 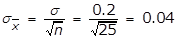delta sub x bar = delta over square root n equals 0.2 over square root 25 equals 0.04. Options A, C and D are incorrect because the numbers given are not derived for the relevant formula.

Domain V—Mathematical Processes and Perspectives

Competency 018—The teacher understands mathematical reasoning and problem solving.

17. Mr. Matthews' precalculus class is studying even and odd functions. Mr. Matthews gave a function to a group of students in his class and asked them to investigate its symmetry without the use of a graphing calculator. Katie, Shayan and Diego are given the function f of x equals x cubed plus x over start absolute value of x end absulute value minus 4, where x is not equal to plus or minus 4. Katie notes that f of 1 equals negative 2 thirds and f of negative 1 equals 2 thirds. Shayan computes f of 3 equals negative 30 and f of negative 3 equals 30. Diego discovers that f of 2 equals negative 5 and f of negative 2 equals 5. After comparing notes, the three students conjecture that the function is odd because the graph exhibits symmetry with respect to the origin. Which of the following activities would enable the group to verify this conjecture?

  1. The students could look for a counterexample by trying several values for x. If they are not able to find a counterexample, they can deduce that the function exhibits symmetry with respect to the origin and is therefore odd.
  2. The students could evaluate the function at an additional eight pairs of values of x that are additive inverses and note that in each case, f of negative x equals negative f of x. They could then deduce that the conjecture is true.
  3. The students could simplify the algebraic expression for f of negative x and establish that it is equal to negative f of x for all values of x, x is not equal to plus or minus 4.
  4. The students could simplify the algebraic expression for f of negative x and establish that it is equal to f of x for all values of x, x is not equal to plus or minus 4.
Enter to expand or collapse answer.Answer expanded
Option C is correct because to establish deductively that the graph of a function is odd and has symmetry with respect to the origin, it suffices to establish that f of negative x equals negative f of x for all values of x in the domain of the function. Options A and B are incorrect because the students may select only points where the conjecture is true and miss points that are counterexamples. Option D is incorrect because it is the criteria for an even function, not an odd function.
Competency 019—The teacher understands mathematical connections both within and outside of mathematics and how to communicate mathematical ideas and concepts.

18. Let C be the set of all right-circular cylinders of radius 3. Let v of x be the volume of the cylinders in C as a function of height, x is greater than or equal to 0. The graph of the function y equals v of x in the xy-plane is

  1. a horizontal line through the point (0, 9 pi).
  2. a line with slope 9 pi and y-intercept 0.
  3. a parabola with vertex (0, 0) passing through the point (1, 9 pi).
  4. a cubic polynomial passing through the points (0, 0), (1, 9 pi), and (3, 27 pi).
Enter to expand or collapse answer.Answer expanded
Option B is correct because the volume of a cylinder varies directly with the height when the radius is kept constant. The resulting graph will be a line with slope open paren 3 squared close paren pi equals 9pi in the first-quadrant of the xy-plane through the origin. Option A is incorrect because the line representing the volume of the cylinder has a non-zero slope of open paren 3 squared close paren pi equals 9pi. Options C and D are incorrect because the volume of a cylinder varies directly with the height when the radius is kept constant and thus is not a polynomial of degree 2 or 3.

19. Bank A and Bank B each offer 5 percent interest rate on savings accounts, but Bank A compounds the interest annually and Bank B compounds the interest semiannually. If Samantha invests 10,000 dollars in a savings account in Bank B, and makes no other transactions in her account, how much more interest will she earn in a year than she would have earned if she had invested the money in Bank A?

  1. 5.00 dollars
  2. 6.25 dollars
  3. 7.25 dollars
  4. 8.50 dollars
Enter to expand or collapse answer.Answer expanded
Option B is correct because if a principal of P dollars is invested at an annual interest rate r, compounded n times per year, and no further withdrawals from or deposits into the account are made, then the future value A after t years, is given by the formula A equals P open paren 1 plus r over n close paren to the power of nt. Because Bank A compounds interest annually, the future value of 10,000 dollars invested in a savings account in Bank A after one year is A equals 10,000.00 dollars open paren 1 plus .05 over 1 close paren to the power of 1 equals 10,500.00 dollars. Bank B compounds interest semiannually, so that the future value of 10,000 dollars invested at Bank B for one year is given by the formula B equals 10,000.00 dollars open paren 1 plus .05 over 2 close paren squared equals 10,506.25 dollars. Thus, Samantha earns 10,506.25 dollars minus 10,500.00 dollars equals 6 dollars and 25 cents more interest in one year by investing in a savings account in Bank B. Options A, C and D are incorrect because they are derived from incorrect applications of the formula.

Domain VI—Mathematical Learning, Instruction and Assessment

Competency 021—The teacher understands assessment and uses a variety of formal and informal assessment techniques to monitor and guide mathematics instruction and to evaluate student progress.

20. Which of the following is a main purpose of formative assessments?

  1. To provide feedback for teachers and students to improve the teaching and learning activities used for instruction
  2. To summarize and record overall achievement at the end of an instructional unit
  3. To evaluate the effectiveness of the curriculum
  4. To identify students ready for promotion to the next level of a course
Enter to expand or collapse answer.Answer expanded
Option A is correct because formative assessments are used at the beginning of, or during, instruction with the aim of refining instructional practices. Thus, they provide feedback to the teachers and students, and are not intended to be reported. Options B, C and D are incorrect because they do not describe formative assessments.

Domain VII—Scientific Inquiry and Processes

Competency 022—The teacher understands how to select and manage learning activities to ensure the safety of all students and the correct use and care of organisms, natural resources, materials, equipment and technologies.

21. The standard deviation of a set of data measurements is related to which of the following? Select all that apply.

  1. Precision
  2. Measurement repeatability
  3. Systematic error
  4. Reproducibility
Enter to expand or collapse answer.Answer expanded
Options A, B and D are correct because the standard deviation is a measure of precision, repeatability or reproducibility. Option C is incorrect because it affects the true or actual value of a quantity (i.e., the accuracy).
Competency 023—The teacher understands the nature of science, the process of scientific inquiry and the unifying concepts that are common to all sciences.

22. Scientific theories are based on which of the following? Select all that apply.

  1. Observations
  2. Experiments
  3. Empirical generalizations
  4. Personal beliefs and speculations
Enter to expand or collapse answer.Answer expanded
Options A, B and C are correct because theories are based on observations, experiments and empirical generalizations. Option D is incorrect because theories are not based on personal beliefs and speculations.

Domain VIII—Physics

Competency 025—The teacher understands the description of motion in one and two dimensions.

23. A ball of mass m is thrown horizontally with an initial speed u sub 0 from the top of a building that is height h above level ground. In the absence of air resistance, what is the speed of the ball when it strikes the ground?

  1. u sub 0
  2. square root 2 g h
  3. u sub 0 plus square root 2 g h
  4. square root of u sub 0 squared plus 2 g h
Enter to expand or collapse answer.Answer expanded
Option D is correct because it correctly accounts for the vector nature of velocity. When the ball strikes the ground, it will have velocity components in both the horizontal and vertical directions. In the absence of air resistance, the external force in the horizontal direction is zero and the external force in the vertical direction is the downward force of gravity. Thus, the horizontal component of the ball's velocity, u sub x, will remain constant throughout the motion at its initial value of u sub 0; that is, u sub x equals u sub 0. Also, the kinematic equations for constant acceleration, y equals 1 half gt squared and v equals gt, can be used to find that the vertical component of the velocity, u sub y, when the ball strikes the ground is equal to u sub y equals square root 2 g h. Thus, the speed of the ball when it strikes the ground is equal to the magnitude of the resultant of the two vectors, or Square root of u sub x squared plus u sub y squared equals square root of u sub 0 squared plus 2 g h. Option A is incorrect because it is equal to the horizontal component of the velocity only. Option B is incorrect because it is equal to the vertical component of the velocity only. Option C is incorrect because it is equal to the sum of the horizontal and vertical components of the velocity, which is not equal to the magnitude of the resultant of the two vectors.

24. Two satellites are each in a circular orbit around Earth at distances R and 2R, respectively, from Earth's center. If the satellite at distance R has an orbital period equal to T, the satellite at distance 2R must have an orbital period equal to

  1. 2 square root 2 T
  2. 2T
  3. T
  4. 1 half T
Enter to expand or collapse answer.Answer expanded
Option A is correct because, according to Kepler's third law, the period T sub 1 equals T at radius R sub 1 equals R is related to the period T sub 2 at radius R sub 2 equals 2R by the equation open paren T sub 2 over T sub 1 close paren squared equals open paren R sub 2 over R sub 1 close paren cubed, which gives open paren T sub 2 over T close paren squared equals open paren 2 R over R close paren cubed equals 8, or T sub 2 equals 2 square root 2 T. Options B, C and D are incorrect because they do not agree with Kepler's third law.

25. A block of mass 2 kg and volume 4 times 10 to the power of negative 4 m cubed is suspended from a thin wire and completely submerged in water. What is the tension in the wire? (Assume g equals 10 m/s squared.)

  1. 0
  2. 4 N
  3. 16 N
  4. 20 N
Enter to expand or collapse answer.Answer expanded
Option C is correct because, according to Archimedes' principle, the buoyant force acting on the block is equal to the weight of the volume of water displaced by the block, or open paren 1,000 kg/m cubed close paren open paren 4 times 10 to the power of negative 4 m cubed close paren open paren 10 m/s squared close paren equals 4 N. The tension in the wire is equal in magnitude to the apparent weight of the block which is equal to its actual weight minus the buoyant force, or 20 N minus 4 N equals 16 N. Option A is incorrect because it results from the assumption that the buoyant force is equal to the actual weight of the block. Option B is incorrect because it results from the assumption that the tension in the wire is equal to the buoyant force. Option D is incorrect because it results from the assumption that the tension in the wire is equal to the weight of the block.
Competency 026—The teacher understands the laws of motion.

26. A 40 kg box is pulled up a long, frictionless ramp by a 400 N force. If the ramp is inclined at an angle of 30 degrees with respect to the horizontal, what is the magnitude of the acceleration of the block? (Assume g equals 10 m/s squared.)

  1. 0
  2. 5 m/s squared
  3. 10 m/s squared
  4. 15 m/s squared
Enter to expand or collapse answer.Answer expanded
Option B is correct because, by Newton's second law, the acceleration of the block is equal to the net force acting on the block divided by the mass of the block. The net force F net on the block is equal to the 400 N force acting upward along the ramp minus the component of the block weight acting downward along the ramp, or
F net equals 400 N minus open paren 40 kg close paren open paren 10 m/s squared close paren sine 30 degrees equals 400 N minus 200 N equals 200 N. So F net equals 200 N.
Thus, by Newton's second law, the acceleration a of the block is equal to a equals F net over m equals 200 N over 40 kg equals 5 m/s squared. Option A is incorrect because a net force is acting on the block along the ramp, which means the block is accelerating. Option C is incorrect because it does not account for the component of the block's weight along the ramp. Option D is incorrect because it results from using the wrong value for the net force acting on the block.

27. A 50 N force is being applied to a 10 kg block on a rough horizontal surface. If the coefficient of kinetic friction between the moving block and the surface is 0.25, what is the magnitude of the acceleration of the block? (Assume g equals 10 m/s squared.)

  1. 2.5 m/s squared
  2. 5 m/s squared
  3. 7.5 m/s squared
  4. 10 m/s squared
Enter to expand or collapse answer.Answer expanded
Option A is correct because the net force acting on the moving block is equal to the applied force minus the force of kinetic friction, or
F net equals 50 N minus mu sub k M g
F net equals 50 N minus open paren 0.25 close paren open paren 10 kg close paren open paren 10 m/s squared close paren
F net equals 50 N minus 25 N equals 25 N . Thus, by Newton's second law, the acceleration of the block is equal to the net force divided by the mass of the block, or F net over 10 kg equals 25 N over 10 kg equals 2.5 m/s squared. Option B is incorrect because it does not account for the frictional force acting on the block. Option C is incorrect because it results from computing the net force as the sum, rather than the difference, of the applied force and the frictional force. Option D is incorrect because it is equal to the acceleration due to gravity, not to the acceleration of the block.
Competency 027—The teacher understands the concepts of gravitational and electromagnetic forces in nature.

28. A simple pendulum of length L has a period T on Earth. If a simple pendulum is to have the same period T on Jupiter, where the gravitational force is 2.5 times as great as the gravitational force on Earth, what does the length of the pendulum have to be?

  1. 2.5L
  2. square root 2.5 L
  3. L
  4. L over 2.5
Enter to expand or collapse answer.Answer expanded
Option A is correct because the period T of a simple pendulum is given by the equation T equals 2 pi square root L over g and, if the period on Jupiter is to be the same as the period on Earth, then it follows that L over g equals L sub Jupiter over g sub Jupiter equals L sub Jupiter over 2.5 g, or L sub Jupiter equals 2.5 L. Option B is incorrect because it results from the assumption that the acceleration due to gravity on Jupiter is equal to square root 2.5 g instead of 2.5g. Option C is incorrect because it results from the assumption that the length is unchanged, which would not give the same period on both planets. Option D is incorrect because it results from the assumption that the acceleration due to gravity on Jupiter is equal to g over 2.5 instead of 2.5g.

29. A planet moves in an elliptical orbit about a star. Which of the following is true about the orbital speed of the planet?

  1. It is constant.
  2. It varies and is greatest when the planet is closest to the star.
  3. It varies and is greatest when the planet is farthest from the star.
  4. It cannot be determined from known physical laws.
Enter to expand or collapse answer.Answer expanded
Option B is correct because, according to Kepler's second law, a planet sweeps out equal areas in equal times in its elliptical orbit about a star, which means that the speed of the planet must be greatest when it is closest to the star. Options A and C are incorrect because they are not in agreement with Kepler's second law. Option D is incorrect because the speed of the planet can be determined from known physical laws.

30. A coil of wire produces a magnetic field of magnitude B. If the number of turns per unit length in the coil is increased by a factor of 2, the magnitude of the magnetic field produced by the coil will be equal to which of the following?

  1. 1 half B
  2. B
  3. 2B
  4. 4B
Enter to expand or collapse answer.Answer expanded
Option C is correct because the magnitude B of the magnetic field produced by a coil of wire with n turns per unit length is given by B equals mu n I, where mu is the magnetic permeability and I is the current in the coil. Thus, increasing n by a factor of 2 will increase the magnitude of the magnetic field by a factor of 2. Options A, B and D are incorrect because they result from assuming the wrong dependence of B on n.
Competency 028—The teacher understands applications of electricity and magnetism.

31. A 4 ohm resistor and a 12 ohm resistor are connected in parallel to a 12 V battery. The power dissipated by the circuit is equal to which of the following?

  1. 48 W
  2. 18 W
  3. 9 W
  4. 4 W
Enter to expand or collapse answer.Answer expanded
Option A is correct because two resistors, R sub 1 and R sub 2, connected in parallel are equivalent to a single resistor given by R sub eq equals fraction R sub 1 R su 2 over R sub 1 plus R sub 2, and, according to Ohm's law, the power P dissipated by the circuit is equal to P equals IV equals V squared over R eq. Thus, R sub eq equals fraction open paren 4 ohm close paren open paren 12 ohm equals fraction 48 ohm squared over 16 ohm equals 3 ohm, which gives P equals open paren 12 V close paren squared over 3 ohm equals 48 W. Option B is incorrect because it results from using the difference of the two resistors as the equivalent resistance to compute the power. Option C is incorrect because it results from using the sum of the two resistors as the equivalent resistance to compute the power. Option D is incorrect because it results from using V instead of V squared to compute the power.

32. Which of the following uses mechanical energy to produce electrical energy?

  1. A transformer
  2. A motor
  3. A generator
  4. A battery
Enter to expand or collapse answer.Answer expanded
Option C is correct because a generator converts mechanical energy into electrical energy. Option A is incorrect because a transformer increases or decreases voltage through electromagnetic induction. Option B is incorrect because a motor converts electrical energy into mechanical energy. Option D is incorrect because a battery converts chemical energy into electrical energy.
Competency 029—The teacher understands the conservation of energy and momentum.

33. A railroad boxcar of mass M is moving at speed u along a straight horizontal track. It collides elastically with a second boxcar of the same mass M that is at rest. What are the magnitude of the total momentum and the total kinetic energy of the two boxcars immediately after the collision?

Momentum Kinetic Energy
A. 0 1 half M u squared
B. Mu 1 half M u squared
C. 0 M u squared
D. Mu M u squared
Enter to expand or collapse answer.Answer expanded
Option B is correct because momentum and kinetic energy are conserved in an elastic collision. The magnitude of the momentum before the collision is equal to the momentum Mu of the moving boxcar, which means that the magnitude of the total momentum of the two boxcars immediately after the collision must also be equal to Mu. The kinetic energy before the collision is equal to the kinetic energy 1 half M u squared of the moving boxcar, which means that the total kinetic energy of the two boxcars immediately after the collision must be equal to 1 half M u squared. Option A is incorrect because the magnitude of the momentum immediately after the collision is not zero (the boxcars are not at rest). Option C is incorrect because immediately after the collision the magnitude of the momentum is not zero (the boxcars are not at rest) and the total kinetic energy is not equal to two times the kinetic energy before the collision. Option D is incorrect because the total kinetic energy immediately after the collision is not equal to two times the kinetic energy before the collision.
Competency 030—The teacher understands the laws of thermodynamics.

34. The second law of thermodynamics is specifically concerned with which of the following?

  1. The conservation of energy
  2. The behavior of gases
  3. The behavior of the entropy of a system
  4. The molecular basis of temperature
Enter to expand or collapse answer.Answer expanded
Option C is correct because the second law of thermodynamics is a statement about the entropy of a system. Option A is incorrect because the first law of thermodynamics, not the second law of thermodynamics, is a statement of the law of conservation of energy. Option B is incorrect because the behavior of gases is a consequence of the kinetic theory of gases, not of the second law of thermodynamics. Option D is incorrect because the molecular basis of temperature is a consequence of the kinetic theory of gases, not of the second law of thermodynamics.

35. When 100 J of heat energy is added to a system, the system does 40 J of work. What is the increase in the internal energy of the system?

  1. 40 J
  2. 60 J
  3. 100 J
  4. 140 J
Enter to expand or collapse answer.Answer expanded
Option B is correct because, by the first law of thermodynamics, the internal energy increase is equal to the difference between the heat added to a system and the work done by the system, which in this case gives 100 J minus 40 J equals 60 J. Option A is incorrect because the increase in the internal energy of the system is not equal to the work done by the system. Option C is incorrect because the increase in the internal energy of the system is not equal to the heat added to the system. Option D is incorrect because the increase in the internal energy of the system is not equal to the sum of the heat added to the system and the work done by the system.
Competency 031—The teacher understands the characteristics and behavior of waves.

36. A light ray passes from air (n = 1) into glass (n = 1.5). If the incident angle is 30 degrees, what is the angle of refraction?

  1. 30 degrees
  2. 60 degrees
  3. sine to the power of negative 1 1 third
  4. sine to the power of negative 1 3 forths
Enter to expand or collapse answer.Answer expanded
Option C is correct because according to Snell's law, n sub air sine 30 degrees equal n sub glass sine theta sub glass, where theta sub glass is the angle of refraction in glass. Thus, 1 half equals 1.5 sine theta sub glass, or theta sub glass equals sin negative 1 fraction 1 third. Option A is incorrect because the refracted ray in the glass must be bent toward the normal, which means that the angle of refraction must be less than 30 degrees. Option B is incorrect because the angle of refraction must be less than 30 degrees. Option D is incorrect because it contains a mathematical mistake.

37. Which of the following is characteristic of light but not of sound?

  1. Dispersion
  2. Constructive and destructive interference
  3. Bending around an obstacle
  4. Propagation in a vacuum
Enter to expand or collapse answer.Answer expanded
Option D is correct because light does not require a medium in which to propagate, whereas sound waves are disturbances in a medium. Options A, B and C are incorrect because all waves, including light and sound waves, exhibit dispersion, constructive and destructive interference and bending around an obstacle.
Competency 032—The teacher understands the fundamental concepts of quantum physics.

38. According to the Bohr model of the hydrogen atom, how does the energy of an electron depend on the principal quantum number n?

  1. The energy of an electron is proportional to 1 over n squared.
  2. The energy of an electron is proportional to 1 over n.
  3. The energy of an electron is proportional to n.
  4. The energy of an electron is proportional to n squared.
Enter to expand or collapse answer.Answer expanded
Option A is correct because, according to the Bohr model, the energy E sub n of an electron in the nth energy level of the hydrogen atom is equal to E sub n equals negative start fraction 13.6 over n squared end fraction e V. Options B, C and D are incorrect because they each have the wrong dependence on n.

39. In the photoelectric effect, light is incident on the surface of a metal and causes photoelectrons to be emitted. For a given metal, which of the following is true about the energy of the emitted photoelectrons?

  1. The energy of the photoelectrons increases when the frequency of the incident light increases.
  2. The energy of the photoelectrons increases when the intensity of the incident light increases.
  3. The energy of the photoelectrons increases when the wavelength of the incident light increases.
  4. The energy of the photoelectrons is characteristic of the metal and independent of the incident light.
Enter to expand or collapse answer.Answer expanded
Option A is correct because increasing the frequency of the incident light increases the energy of the emitted photoelectrons. Option B is incorrect because increasing the intensity of the incident light increases the number of emitted photoelectrons but has no effect on the energy of the photoelectrons. Option C is incorrect because decreasing, not increasing, the wavelength of the incoming light increases the energy of the emitted photoelectrons. Option D is incorrect because the energy of the emitted photoelectrons is not independent of the incident light.

Domain IX—Science Learning, Instruction and Assessment

Competency 033—The teacher understands researched-based theoretical and practical knowledge about teaching science, how students learn science and the role of scientific inquiry in science instruction.

40. Which of the following student responses is an example of correct conceptual understanding?

  1. The mass of an object is equal to its weight.
  2. The speed of light is greater than the speed of sound.
  3. Heavy objects always sink in water.
  4. The Moon and the Sun are the same size.
Enter to expand or collapse answer.Answer expanded
Option B is correct because the speed of light is much greater than the speed of sound. Option A is incorrect because mass is a measure of the amount of matter an object contains, while weight is the force of gravity on an object. Option C is incorrect because some heavy objects can float on water. Option D is incorrect because the Sun is much larger than the Moon.